Orsay: Why are we using position-sensitive strip detectors, again?

...besides the logistical "that's what we have on hand to fry" reasons, I mean--which in themselves can be pretty compelling.

While poking around looking for the best way to arrange the PSSSD detectors (on which more anon), I got to thinking how nice it would be to have something that gave full coverage at small angles. Then I got to thinking, well, maybe we need the extra-good position resolution that the PS3D detectors provide. Then I figured, hey, why not test that assumption. So here's the results: Q calculated for the 6.404 MeV level in ¹⁸O, various different ways: 1. using the true value of θ; 2. using the true values of both θ and φ; 3. using the value of θ that the PS3D gives us; 4. using both θ and φ from the PS3D. (Using θ and φ measured for the alphas you calculate x, y, and z momentum components for the residual nucleus and then use that to calculate its energy and hence Q; using just θ you calculate the parallel (to the beam direction) and perpendicular components of its momentum.)



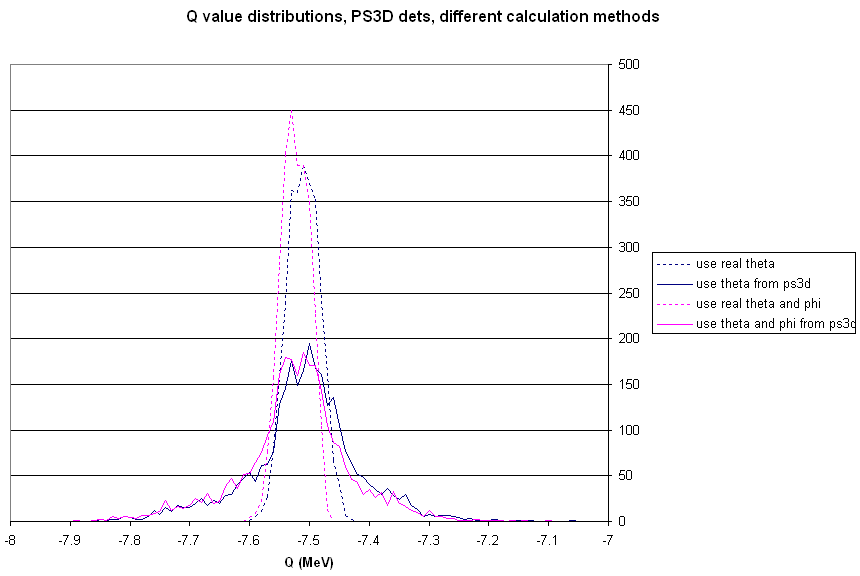
What you see is that it makes no difference whether you use φ to calculate Q or not. This suggests that the bad φ resolution of LEDA or S2 detectors wouldn't be a problem. Check it out.



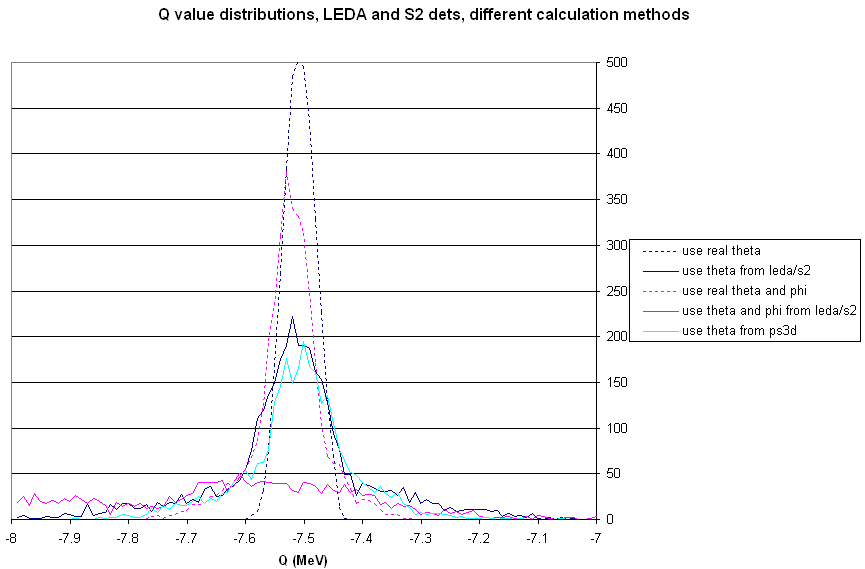
When you calculate Q with LEDA/S2 θ information, you get (essentially) the same result as from the PS3Ds, even though if you include φ, the resolution is crap. For comparison, the PS3D θ result is shown on the same plot.

Conclusion: Using concentric-strip detectors in this case doesn't limit the quality of the information we get. There's a chance that it might improve the quantity too. Stay tuned for the next entry...