17O(p,α) proposal: detector positions and efficiency

These are just relkin calculations for 3.3 MeV 17O on a 4.5 Torr H gas target. I make the energy loss over 1 cm of target to be 13 keV. I did the kinematics for the reaction and the elastic scattering at the beginning and end of that range (i.e. 3.3 MeV at 0 cm, 3.287 MeV at 1 cm). The figures are
1. an e vs theta plot: the particle energies (neglecting energy loss/straggling of products in target and in dead layers) vs the real god-given particle angles (again obviously neglecting angular straggling in the target). The lines for the same reaction at the two positions lie practically on top of each other.
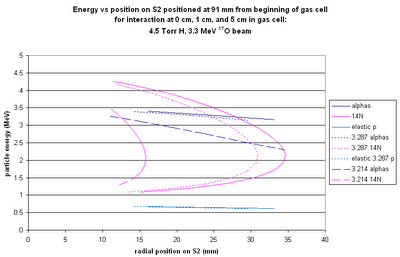
2. an e vs S2 position plot. I put an S2 at 91 mm downstream from the 0 cm position, and calculated the radial position of the particles on it, if they hit it. (The position was chosen to maximize the number of 14N we catch: we throw away about 25% of the small-angle 14N with this position, but we'd have to throw away 5% anyway, and it may turn out that this is the optimal position.)
3. an e vs "barrel" position plot. I put a cylindrical barrel of arbitrary length at a radial distance of 40 mm from the beam line, with a starting position somewhere near the S2 (position increases upstream in this plot, so something going off from 0 cm at 90' would be at ~100 mm on the barrel).

Next step: figure out what this means: by detecting the alpha and 14N in some sort of configuration like this, can we deduce the centre of mass energy?

Comment from Tom: putting an S2 inside the dragon gas cell would indeed involve making a new target box. (We're cunning like that.)

A note on efficiency: with an S2 positioned as indicated, we catch 75% of the 14N from the reaction at the 0 cm position. With a 10 cm long cylindrical barrel detector, we catch 82% of the alphas, with an additional 10% going into the S2. The total efficiency is then about 71%. If the barrel detector has less than 100% coverage over its angular range, the efficiency will be decreased by a corresponding amount--for example 50% coverage (like we might get with two rectangular detectors if we were very cunning) leads to 35% total efficiency, whereas with four we might get about 60% total efficiency.

A further note on the figures: the ones I've just uploaded include the effects of reactions at 5 cm as well as at 0 and 1 cm--just to see.