18Ne(d,p)

Alison says:

18Ne beam at 54 MeV with 8mm (probably FWHM) beam spot on CD2 target of 410 ug/cm2 thickness.
Detecting protons from (d,p) at backangles with LEDA at 74mm.
want to know resolution of protons, taking into account beam straggling, dead layer, angular width of strip and straggling, everything basically
excited states at 2.5 MeV, 4.033MeV, 4.140MeV and 4.329 MeV

Here's some results...
Simulated energy spectra for the innermost, middle, and outermost strips of the upstream LEDA:

When I try fitting a gaussian to the lowest energy peak in strip 0, I end up with a sigma of something like 300 keV.
Energy vs effective angle of strip: colours and sizes of markers indicate number of hits:

Orsay: simulate elastic scattering

80 MeV ¹⁴C on carbon



40 MeV ¹⁴C on carbon



80 MeV ¹⁴C on gold



40 MeV ¹⁴C on gold



These simulations include energy loss/straggling in the target and dead layer, along with the detector's energy resolution.
Bottom line: the gold runs should provide well-separated lines in det 1 and nothing at all in quad (so ignore it; no requirements at all on goodness-of-events in the quad), while the carbon events should be coincidences between det 1 and quad--maybe initial energy calibration of det 1?

Orsay status report

Two types of progress....

1. Data analysis.

• I have a working sort code, with various bells and whistles, including the ability to count the number of "good strips"--if a strip in det 1 has a good energy signal in both ends, the good strip count for det 1 is incremented. Similarly for the quad.
  
• I have reasonable gain-match parameters for detectors 2,3, and 4. Detector 5 may not have been biassed, and the gain-matching parameters look dodgy. These parameters are derived from the alphas--since we irradiated only the quad, the only way to gain-match detector 1 will be elastic scattering.
  
• I sorted the data for the alpha run and extracted the energy spectra of all the individual strips. Here's the results, for several of the better strips for one of the better detectors...
  

The energy spectra for several strips are displayed (jaggedy coloured lines) together with triple-Gaussian fits (red smooth lines) courtesy of Igor. X axis is channel number, not keV. (These spectra are for an intermediate stage in gain-matching, and were used to obtain the final parameters: the parameters were adjusted so that the centroids of the fitted peaks were all the same.) What emerges from these spectra is that the detectors have an energy resolution with σ=110 keV.
UPDATE: Here are simulations done using that value for the detector resolution, compared with a few of the better strips' data. The agreement is decent.

• The actual data...well, various different ways of sorting are presented pell-mell in this previous blog entry. Basically, the most conspicuous feature in the quad energy-position spectra is either noise or an artefact of the joining boards. As to which sorting option is the best, see...
  

2. Simulations and other calculations

• I've got a geometric code for my monte-carlo sims that reproduces (to good-enough accuracy) the final positions of the detectors. (It characterises their positions in terms of the radial distance and the thetaφ angles of their centres--thus omitting the slight skew-ness of all of the quad detectors.)
  
• the most basic kinematic calculations, done using jrelkin and neglecting all energy loss/straggling effects:
  

Energy vs. angle curves for all the elastic scattering reactions we did. (click for a larger version.) The dotted lines represent the energy/angular ranges of the detectors (det 1 and quad).


Angle-vs-angle curves: the angle of the scattered beam particle is plotted against the angle of the knocked-out target particle. The dashed boxes represent the areas where both particles' angles take them towards a detector....

...but the above graph doesn't really tell the whole story about what's a coincidence and what's not. In order for an elastic scattering event to give a coincidence, not only must both particles go in the appropriate directions, but they must also both have energies within the range of the detectors.
Update:


(sorry about the random change of sign on the x axis.) The above plot was made using cunning energy requirements on both particles: if a particle's angle is less than 40', its energy must be between 1 and 100 MeV, but if its angle is greater than 40', its energy must be between 1 and 25 MeV. The lines are drawn for the cases where those conditions are met for both particles and so the energies of both particles are such that coincidences can be detected. The lines that fall within the boxes indicate when the angles of both particles permit coincidences.
What this graph seems to indicate:

• There are no ES coincidences from the gold runs at either energy
• For the 12C and 13C runs, we don't lose much of the data by requiring coincidences
• There are absolutely no coincidences for which either particle is above 90', so no way of using ES data to get a position-to-angle measurement for detectors 2 and 3. It should, on the other hand, be possible to calibrate dets 1 and 4 and maybe 5.
  

Other things I'm checking out:

• the total efficiency of the detectors: both percent of solid angle and efficiency for detecting a triple coincidence from the reaction, taking into account the energy thresholds
• the resolution we can expect in the Q value we calculate

...but the most urgent question is really the one about the elastic scattering: if we can identify ES loci in all the detectors, we can confirm that the gainmatching parameters are good, and we can maybe get some kind of position-on-detector to theta calibration. So far it's clear that there is stuff present, at least in dets 1, 4, and 5 (dets 2 and 3 seem mostly to show randoms), but I haven't yet managed to identify any of it.
The way forward: do simulations quickly for a few elastic scattering cases, to give me an idea of what I'm looking for in the data; then move on to sorting actual runs. Use as a condition that there be at least one good strip in both the quad and det 1; also veto quad events on the complementary strip's signal, to get rid of the weird top features. (That's for the carbon(12/13) targets; for the gold target require at least one good strip total because we don't expect real coincidences.)

18F(d,p): various factors affecting resolution

The idea: use an ¹⁸F beam of some energy between 14 and 100 MeV on deuterated polyethylene target of some thickness between 10 and 100 μg/cm²: the reaction is ¹⁸F(d,p)¹⁹F*(α)¹⁵N. Detect either just the protons or all three final particles (p, α, ¹⁵N). Calculate the Q value corresponding to the final energies and deduce the excitation energy of ¹⁹F. See whether we can resolve the 6.497, 6.528 MeV levels. Because of the beam's energy loss and straggling through the target, using the proton's final energy to calculate Q might not give very accurate results, whereas the alpha's energy might be a more precise indicator of ¹⁹F's energy.

The input:

• 90 MeV ¹⁸F beam (corresponds to optimum beam energy of 5 MeV/u)
• 20 μg/cm² DH₂ target
• detectors with arbitrary granularity (i.e. pixel size): take the S2 as a starting point: test the effect of changing the theta (annular) strip pitch from 0.5 mm to 1 mm; test the effect of having 16 or 32 phi (radial) strips.
• energy/angular straggling parameters derived from SRIM

Things I've left out

• energy spread of the beam entering the target
• exact cross section (have assumed isotropic distribution in the centre of mass frame).
  

Test, independently, all the different possible contributions to the final Q resolution:

• energy loss/straggling and angular straggling of beam through the target
• energy and angular straggling of the outgoing particles through the target (assume we can reconstruct the energy perfectly, so count the energy straggling but not the energy loss)
• energy straggling of particles in the detectors
• energy resolution of detectors
• beam spot size
• granularity of detectors

Doing the calculations

• Monte Carlo simulation of reaction
• use relativistic energy/momentum to calculate Q (n.b. this is different from e.g. the LLN experiment, where non-relativistic energy/momentum were good enough)

Results
(click on the image for a larger version)

What the graph shows: the standard deviation of the Q values calculated using each different method.
"Qp" means the Q value calculated using only data from the protons; "Qa15" means that only data from the alpha and ¹⁵N are used; Qcalc means that a complicated bit of algebra is done to eliminate the proton and ¹⁵N energies and to reconstruct the beam energy before the reaction.
the different cases:

• "perfect": no energy losses: Q is calculated using the initial energies/angles of all particles
• "beam in target": the reaction takes place at a randomly chosen depth in the target, and uses the beam's modified energy/angle at that point
• "detector energy resolution": see what effect the detector's resolution has: add a gaussian with 15 keV fwhm to a particle's energy
• "particles in target": calculate energy/angle straggling of outgoing particles through the target
• "particles in deadlayer": calculate energy straggling of particles in the deadlayer: assume that the detector is flat and perpendicular to the beamline
• "particles in deadlayer, incidence angle = 0": same as above, only assume that the particles always hit the detectors square on.
• "beamspot size": assume that the beam has a circular profile, with gaussian shapes in the both x and y directions, with σ=1 mm: calculate the precise position of the particles on the detectors, 500 mm down- and upstream.
  
• "detector granularity (standard)": the detectors have 0.5 mm strip pitch and 32 strips in φ,are of infinite extent, and are located 500 mm down- and upstream: calculate the effective position of the particles on the detectors: i.e. the location of the middle of the pixels that the particles hit.
  
• "detector granularity: 16 phi strips": decrease the number of phi strips
• "detector granularity: strip pitch 1 mm": increase the strip pitch

The clever algebra method, Qcalc, does indeed do a better job than the α+¹⁵N method in accounting for the beam energy loss in the target, but that's about its only strength. In general it is decent when it comes to things that mostly affect particles' energies, but it's very sensitive to the particle's angles, so it can't handle granular detectors: the Q values for the realistic detectors range between positive and negative infinity.
The α+¹⁵N method isn't as bad as Qcalc for the granular-detector cases, but it's still pretty bad.
For Qp, the sizes of the spreads in Q introduced by the various factors are all roughly on the same order. They also all depend on the angle of the protons: in some cases they're stronger at backward angles, and in some cases weaker.

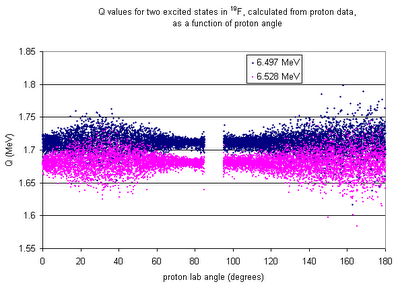
Calculate Qp, using all factors combined, and see what the total angular dependence is.
Input: detectors are perpendicular to the beam line, located at 500 mm from the target, and have 0.5 mm theta strip pitch and 32 phi strips; the beam has σ=1 mm; Q is calculated using (proton energy = initial energy + straggling through target + straggling through dead layer + detector resolution factor) and (proton angle = initial angle + angular straggling through target) and (beam energy = average beam energy half way through the target).
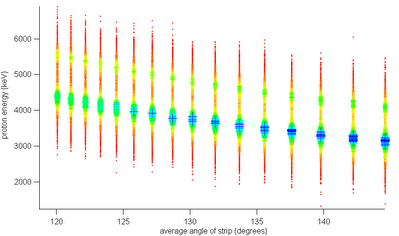
Results: Q value as a function of angle, for the two states of interest...

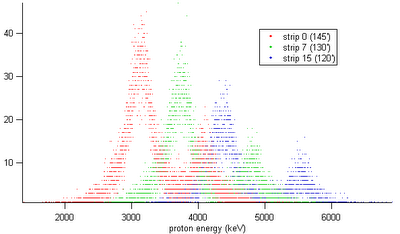
Distribution of Q values for high-angle protons (i.e. theta > 120')

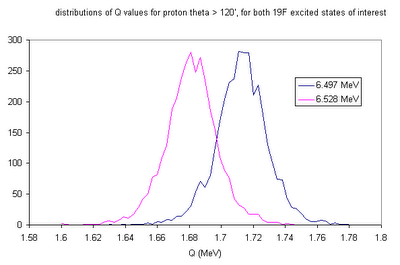
It looks like it's at least possible in theory to make this measurement!